Research interests
I am interested in the connections and interplay between mathematical physics, topology, representation theory, and (higher) category theory.
My research interests include:
- topological and conformal field theories
- quantum algebra (Hopf algebras, tensor categories, module categories, ... )
- defects and categorical symmetries in quantum field theories
In my PhD project I am studying surface defects in finite non-semisimple three-dimensional topological quantum field theories with the goal of applying
them in a holographic setting to describe properties of two-dimensional conformal field theories which appear as boundary theories of such 3d TQFTs.
If you are interested in my research, I am happy to chat or give seminar talks, so please contact me. I am currently on the job market!
Publications
Preprints
Simons Lectures on Categorical Symmetries, edited by Michele Del Zotto and Claudia Scheimbauer.
Modular functors from non-semisimple 3d TFTs, with Ingo Runkel
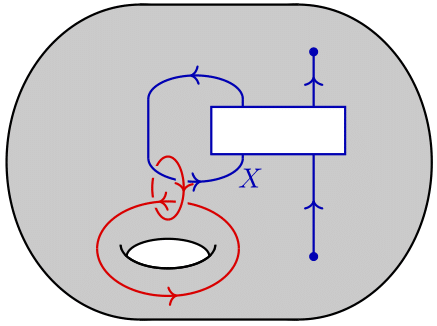
Modular functors arise in the rigorous study of two-dimensional CFTs and are traditionally defined as systems of projective representations of mapping class groups
of surfaces that are compatible with the gluing of surfaces. There are several well-known constructions of modular functors. For example the holographic approach
via three-dimensional TFTs of Reshetikhin-Turaev type which uses a finite semisimple modular tensor category as input datum. In the 90ies Lyubashenko gave a
construction that no longer requires semisimplicity of the input category and recovers the RT-construction in the semisimple case.
It is thus natural to wonder if Lyubashenko's modular functor can be obtained from a three-dimensional TFT also in the non-semisimple case.
In this article we use the 3d TFTs constructed by De Renzi et al from a not necessarily semisimple modular tensor category C to answer this question affirmitively.
To be a bit more precise we construct a modular functor as a symmetric monoidal 2-functor from a bordism 2-category to a 2-category of linear categories and show that
the gluing morphisms coincide with the ones of Lyubashenko.
We also discuss how pulling back the modular functor for C to a 2-category of bordisms
with orientation reversing involution cancels the gluing anomaly, and we relate this pullback to the
modular functor for the Drinfeld center of C. Finally, we also discuss the connection to the 2-category of open-closed bordisms and the corresponding modular functors.
Master's thesis
TQFTs with additional structure, University of Vienna, 2022
In my Master's thesis I studied topological quantum field theories (TQFTs) with defects and tangential structures.
In the first part of this project, I focused on finding a suitable definition for a general n-dimensional bordism category
where all manifolds are stratified, and all strata come with a fixed type of tangential structure. In the second part,
I focused on the concrete case of 2-dimensional theories defined on bordisms with spin structures in more detail.
The main goal here was to construct a 2-category out of a given TQFT, which contains as much information about the TQFT
as possible. I found that such a construction indeed works, and the resulting 2-category comes equipped with a
2-endofunctor which squares to the identity. This 2-endofunctor has a topological origin as it comes from the
non-trivial deck transformation on the Spin bundles.
